Board games have been a staple of family entertainment for generations, but beneath their colorful boards and quirky pieces lies a world of mathematical wonder. From the probability calculations in Monopoly to the geometric patterns in Blokus, these games are secretly teaching us complex mathematical concepts. As we roll dice, move pieces, and strategize our way to victory, we’re unknowingly engaging with principles that mathematicians have studied for centuries. Uncovering these hidden mathematical gems can give us a whole new appreciation for our favorite pastimes and might even improve our gameplay. So, grab your favorite board game and prepare to see it through the lens of a mathematician!
Probability in Monopoly

Monopoly, the classic property trading game, is a masterclass in probability theory. The distribution of dice rolls affects the likelihood of landing on certain properties, influencing strategic decisions. For example, the properties 6-9 spaces from jail are landed on more frequently due to the higher probability of rolling those numbers with two dice. Understanding these probabilities can give players a significant advantage in property acquisition and development strategies.
Geometry in Blokus

Blokus is a game that revolves around geometric shapes and spatial reasoning. Players must fit their pieces onto the board, touching only at the corners. This gameplay mechanic is a practical application of polyomino theory, a branch of mathematics that studies geometric shapes formed by connecting squares. The game challenges players to visualize and manipulate these shapes in ways that maximize their coverage of the board while blocking opponents.
Game Theory in Chess

Chess is often considered the ultimate game of strategy, and it’s a perfect example of game theory in action. Each move a player makes influences their opponent’s available options, creating a complex web of decision-making. The concept of “minimax,” where players try to minimize their maximum possible loss, is a fundamental principle in both chess strategy and mathematical game theory. This principle extends far beyond the chessboard, finding applications in economics, political science, and artificial intelligence.
Set Theory in Set

The card game Set is a rapid-fire exercise in recognizing patterns and applying set theory principles. Players must identify sets of cards where each feature is either all the same or all different. This game directly relates to the mathematical concept of set theory, which deals with collections of objects. By playing Set, players are unknowingly practicing the same logical thinking that mathematicians use when working with complex set operations.
Combinatorics in Scrabble

Scrabble might seem like a game purely about vocabulary, but it’s deeply rooted in combinatorics, the branch of mathematics dealing with combinations of objects. Players must consider the possible combinations of letters to form words, while also strategizing about board positions. The game involves calculating probabilities of drawing certain letters and optimizing word placements for maximum points, making it a playground for combinatorial thinking.
Graph Theory in Ticket to Ride

Ticket to Ride, a game about building train routes, is a colorful implementation of graph theory. The game board represents a graph, with cities as vertices and train routes as edges. Players unknowingly use concepts like shortest path algorithms and network flow as they plan their routes. The strategies employed in Ticket to Ride mirror the same principles used by mathematicians and computer scientists to solve complex network problems.
Topology in Go

The ancient game of Go is a beautiful example of topological concepts in action. As players place stones on the board, they create territories and enclosures, dealing with concepts like connectivity and surrounded regions. These ideas are fundamental in topology, a branch of mathematics that studies properties of space that are preserved under continuous deformations. The strategic depth of Go comes from these topological considerations, making it a favorite among mathematicians and computer scientists.
Number Theory in Yahtzee

Yahtzee, a dice game of chance and strategy, is secretly teaching players about number theory and combinatorics. The scoring system requires players to group numbers in various ways, such as finding pairs, straights, or collecting all of one number. This process of categorizing and optimizing number combinations is similar to the work done in number theory, particularly in areas like partition theory and combinatorial number theory.
Linear Algebra in Battleship
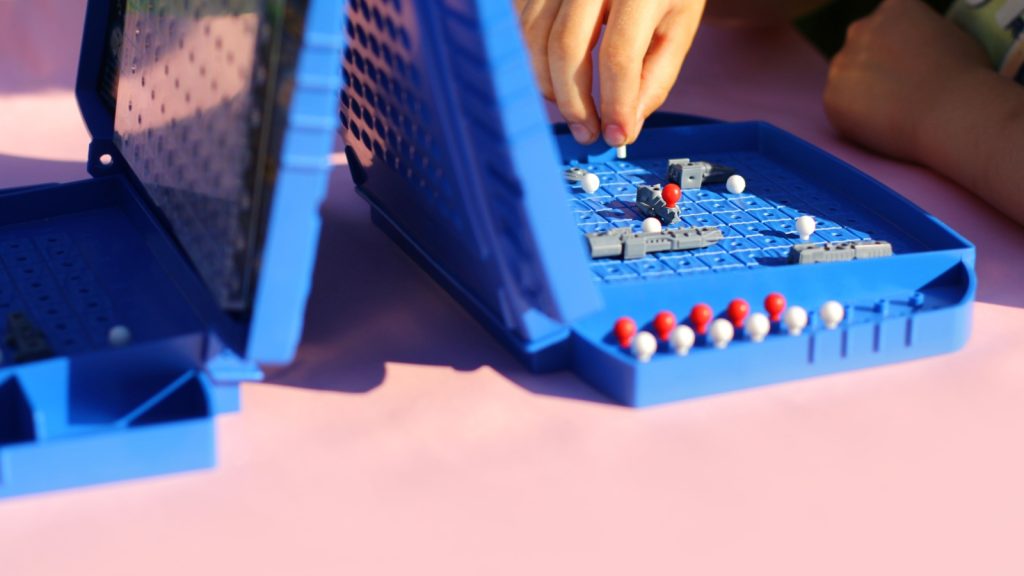
The classic game of Battleship is essentially an exercise in coordinate geometry and matrix operations, key concepts in linear algebra. Players use a grid system to track their guesses and their opponent’s ships, effectively creating and manipulating matrices. The optimal strategies for Battleship involve probability calculations and pattern recognition, skills that are fundamental in more advanced linear algebra applications.
Optimization in Catan

Settlers of Catan is a game of resource management and strategic development that mirrors many real-world optimization problems. Players must optimize their placement of settlements and roads to maximize resource collection and minimize blockages. This type of decision-making process, where players must balance multiple competing factors to achieve the best outcome, is a practical application of optimization theory, a crucial area of mathematics used in fields like operations research and economics.
Fractals in Carcassonne

The tile-laying game Carcassonne creates landscapes that often resemble mathematical fractals. As players build cities, roads, and fields, they’re unknowingly creating self-similar patterns that repeat at different scales. This property of self-similarity is a defining characteristic of fractals, a concept in mathematics that has applications in fields ranging from computer graphics to the study of natural phenomena like coastlines and snowflakes.
Chaos Theory in Risk

The game of Risk, with its global conquest theme, is an unexpected showcase of chaos theory principles. Small initial differences in player decisions can lead to vastly different game outcomes, a phenomenon known as the “butterfly effect” in chaos theory. The complex interactions between players’ strategies, dice rolls, and territorial control create a system that’s highly sensitive to initial conditions, much like the chaotic systems studied by mathematicians and physicists.
16 American Foods Brits Can’t Stand

Food preferences can vary wildly across cultures, and what’s considered a delicacy in one country might be seen as downright bizarre in another. This cultural divide is particularly evident when comparing American and British cuisines. While the two nations share a common language, their tastes in food can be worlds apart. From overly sweet concoctions to processed cheese products, there are certain American foods that many Brits find hard to stomach.
Read More: 16 American Foods Brits Can’t Stand
Katy Willis is a writer, master herbalist, master gardener, and certified canine nutritionist who has been writing since 2002. She’s finds joy in learning new and interesting things, and finds history, science, and nature endlessly fascinating.

